This is a website of Prof. Kazushi Ahara, Meiji university, Japan

List

  • quasisphere(quasi-fuchsian 3d fractal)
  • hyperbolic: papercrafts
  • hyperbolic: 3D printed objects
  • hyperbolic: wammycrafts
  • hyplane: diamond lattice
  • hyplane: cubic lattice
  • hyplane: bird tree
  • hyplane: goldfish
  • Energy of a knot

    • quasisphere(quasi-fuchsian 3d fractal)

    What's quasi-sphere?

  • A quasi-sphere is a kind of fractal shapes in the three dimensional space. This shape is derived from a quasifuchsian group in a pure mathematical way. We have many pictures of quasi-sphere, which are derived from sphairahedra and inversions. Dr. Y. Araki and I construct many examples of sphairahedra in our paper. These fractal figures are the first images in the world.

    • What's sphairahedron?

  • A sphairahedron is a new word made from two words, ‘sphaira-‘ (=spherical) and ‘-hedron' (=polyhedron). Usually we consider a polyhedron as an area surrounded by planes. A sphairahedron is an area surrounded by spheres or planes. (We may regard a plane as a kind of spheres, because a plane is a sphere thru the infinity point.)
  • A sphairahedron has faces, edges and vertices as a polyhedron does. That is, each sphere is called a face. Each intersections of two faces is called an edge. Each intersection of three (or more) edges is called a vertex.
  • A sphairahedron is called regular if the dihedral angel of each edge divides 180 degree. (For example, 90 degree, 60 degree, 45 degree, and 30 degree.)
  • sphairahedron is called ideal if for each vertex, three (or more) edges are mutually tangent at the vertex.

    • Reference

  • [1] Ahara K. and Araki Y., “Sphairahedral Approach to Parameterize Visible Three Dimensional Quasi-Fuchsian Fractals”, CGI2003

    •

    hyperbolic: papercrafts

    hyperbolic truncated icosahedron

    hyperbolic truncated dodecahedron

    hyperbolic: 3D printed objects

    hyperbolic: wammycrafts

    hyplane: diamond lattice

    hyplane: cubic lattice

    hyplane: bird tree

    （（７，７，７）ハイプレインの上のメタモルフォーゼです．）（2001年）

    hyplane: goldfish

    擬似双曲面（負定曲率曲面）の上に描かれた金魚の充填図柄です。金魚の尻尾のところに注目すると、６匹の金魚の尻尾が集まっている場所と、７匹の金魚の尻尾が集まっている場所があることがわかります。つまり、この充填は６対称性と７対称性を同時に持ち合わせていることがわかります。

    フェーズ１（残暑見舞いのはがき仕様です。が、これを投函しても届かないでしょう。）




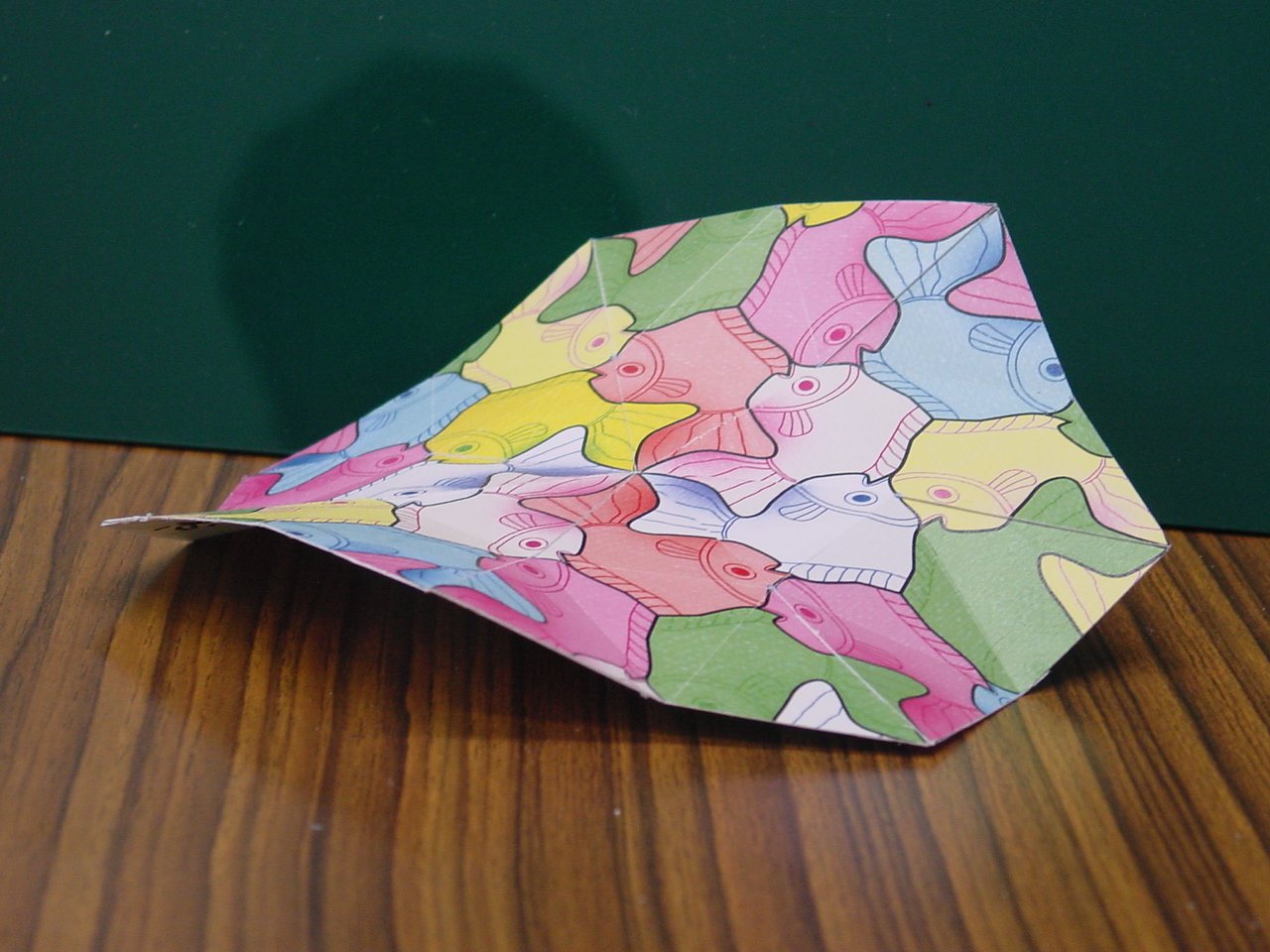
    フェーズ2

    Energy of a knot

    輪の3次元埋め込み（結び目）に，２次静電エネルギーを考えて安定状態をシミュレーションしたものです．（１９９０年．）PC-9801RAというパソコン１台で作りましたので，とても手間暇がかかりました．それだけに愛着もあります．

    ↑ PAGE TOP